Redefine Math Education with Building Thinking Classrooms

Reflecting on my early education, I remember math class as a place where we were expected to sit in neat rows, focus solely on our own papers, and strictly apply the strategies our teachers demonstrated. Asking questions during the lesson was seen as a sign of inattention. Seeking help from classmates was labeled as “cheating,” even standing up from our chairs could prompt a reprimand. Does this sound familiar?

In his book, Building Thinking Classrooms in Mathematics Grades K-12, author Peter Liljedahl describes these structures as “institutional norms–norms that have not changed since the inception of an industrial-age model” that shape students’ behavior from the early years of education (Liljedahl, 2021).

As I embarked on my journey with Building Thinking Classrooms, I couldn’t help but recall my experiences as that timid student who followed the rules and kept her ideas to herself. My math education was limited by the boundaries set by rigid institutional norms in the math setting. It made me wonder if I had imposed those same limitations on students in my math classroom.

At the heart of Liljedahl’s vision lies the concept of the “Thinking Classroom” – a dynamic ecosystem where mathematical discourse thrives and students engage in productive struggle.

It’s not just a set of strategies. It’s a comprehensive approach to building a classroom environment where thinking takes center stage.

The Story of Jane

In his book, Liljedahl begins with Jane’s story. Jane, a dedicated middle school mathematics teacher, cares deeply about her student’s success. She commits to preparing them for the next grade level.

Jane contacted Liljedahl because she wanted to use effective problem-solving tasks in her classroom. Liljedahl selected a few successful thinking tasks from his classroom experience and observed Jane as she implemented these with her students.

Despite her best efforts, Jane struggled to engage her students effectively. Below is an example of one of the thinking tasks Liljedahl shared with Jane:

As I read the introduction to Liljedahl’s Building Thinking Classrooms book, I couldn’t help but imagine my teachers tackling similar tasks with my classmates and me. Liljedahl observed that Jane’s class exhibited frustration and non-thinking behaviors when given thinking problems, such as the Cats and Rats task. Jane’s students waited for her to step in, do the thinking for them, and demonstrate the “right way” to solve the problem. 

As a young child molded by normative structures, I would likely have waited for the teacher to demonstrate how to solve the problem instead of engaging in productive struggle, just as Jane’s students did. How do you think you would’ve done with this task? How do you think your students would do with this task?

Recurring Patterns in Classrooms

Intrigued by his observations in Jane’s class, Liljedahl investigated whether this issue was apparent in other classrooms. He observed 40 well-regarded teachers across 40 different schools. He discovered a recurring pattern: students were not engaging in critical thinking but were instead mimicking their teachers during “now-you-try-one” tasks commonly known as “I do, We do, You do” tasks. This led students to have short-term success and a lack of problem-solving autonomy.

Liljedahl concluded the problem was not isolated to specific schools. But was, in fact, a systemic issue affecting education broadly. Drawing from cognitive science, he highlights the importance of:

• interaction
• dialogue, and
• reflection in nurturing deep understanding and problem-solving skills among students.

What’s the Connection?

Is there truly a connection between institutional norms and non-thinking behaviors?

Liljedahl and Darien Allen tackled this question by conducting a study focused on student behaviors during “now-you-try-one” activities. Similar to Jane, for many of the teachers they observed, now-you-try-one tasks appeared to be part of the fabric of what it means to teach in a math classroom (Liljedahl & Allen, 2013a).

A "now-you-try-one-task" is a task that teachers ask students to do after the teacher has demonstrated to students how to do something. Now-you-try-one tasks are a type of self-assessment where students and teachers learn whether the demonstrations were a success.

For example, a teacher might demonstrate how to regroup when adding two-digit numbers. After working through a few examples, the teacher asks students to solve a similar problem independently. Then, the teacher waits for four minutes and 22 seconds. This is the average time teachers give students to do a now-you-try-one task before they go over how to solve it. Then, in many cases, teachers give the students another now-you-try-one task. Again, does this sound familiar?

If students aren’t thinking in the math classroom, what are they doing?

Non-Thinking Behaviors

Liljedahl and Allen observed that many students were not genuinely engaged in thinking. Instead, they exhibited non-thinking behaviors such as slacking, stalling, faking, and mimicking:

• Slacking: Students who were disengaged or uninterested often using excuses like going to the restroom or sharpening their pencils to avoid the task.
• Stalling: Students who admitted they didn’t understand the task and hoped that by waiting, the teacher would eventually provide the solution.
• Faking: Students who appeared to be on-task but were actually waiting for the teacher’s assistance, similar to students who were stalling.
• Mimicking: Students who could solve problems that closely resembled the examples given by the teacher but struggled with tasks requiring independent thought.
• Tried It: Students who attempted to reason through the task independently, whether they were correct or incorrect.

Interviews with students revealed that while stallers used off-task behaviors to mask their confusion, fakers pretended to be engaged until the teacher intervened. Mimicking students succeeded with familiar tasks, leading to short-term success, but faltered when faced with new challenges requiring the application of conceptual mathematical knowledge.

Alarmingly, Liljedahl found that in a typical one-hour lesson, 75% to 85% of students exhibited non-thinking behaviors throughout the entire period. In contrast, the remaining students who tried it independently engaged for only eight to 12 minutes.

The Rise of Building Thinking Classrooms

Over the next 15 years, Liljedahl worked with over 400 K-12 teachers to move beyond superficial engagement and foster genuine thinking. He aimed to “deliberately break institutional normative structures” to increase the number of students actively thinking and the time spent doing so (Liljedahl, 2021).

Liljedahl challenged long-established institutional norms by experimenting with 14 variables that impact classroom thinking. These “contrarian design principles” eventually proved to be more effective than traditional practices, emerging as “optimal practices for thinking” (Liljedahl, 2021).

In his study, a group of teachers tested each practice for two weeks. If successful, the practice was introduced to other educators in different settings with different student demographics. Practices demonstrating improvements in student thinking behaviors were implemented in a longitudinal study with another group of teachers for six to eight weeks.

They established practices that consistently proved effective as optimal for thinking in the classroom.

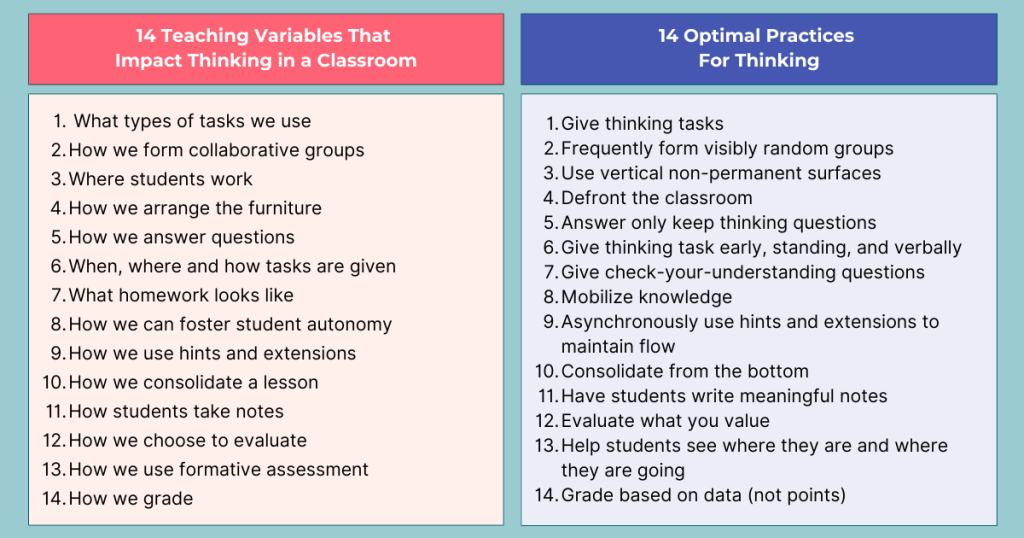
What This Means for Math Classrooms

So, what does this mean for our teaching practices and student accountability in math classrooms?

The research is compelling, but how feasible is it to implement all 14 optimal strategies while covering an entire year’s content within a limited timeframe? Strategies like frequent visibly random grouping, vertical non-permanent surfaces, and rich tasks can transform the classroom into a dynamic environment where every voice is valued and mistakes are embraced as learning opportunities.

Conclusion

I’m excited to embark on this journey with you as we delve into how disrupting traditional institutional norms can make our math classrooms more interactive, engaging, and empowering. The 14 optimal practices are organized into four toolkits. We will explore these in our series of Building Thinking Classrooms blogs.

By adopting these practices, we encourage our students to become architects of their own learning. As Peter Liljedahl aptly puts it, “The classroom is no longer a place where students come to watch teachers work; it is a space where everyone comes to think, and by thinking, transform themselves and the world around them.”

The Math Team at ESC Region 13 offers a robust list of workshop opportunities throughout the year. Visit our workshop catalog to find a course that is right for you. Keep up with the latest math news and developments by subscribing to our newsletter. Check out our other blog posts. And don’t forget to bookmark our math webpage!

---

Resources

• Liljedahl, Peter (2021).Building Thinking Classrooms in Mathematics Grades K-12. Corwin.
• Liljedahl, P. & Allan, D. (2013a). Studenting: The case of “now you try one”. In Lindmeier, A. M. & Heinze, A. (Eds.). Proceedings of the 37th Conference of the International Group for the Psychology of Mathematics Education, Vol. 3, pp. 257-264. Kiel, Germany: PME.